Self-Projective sets
Keywords: Self-Projective sets , Fractals , Semigroups , Matrices , Zeta-functions , Iterated Function Systems
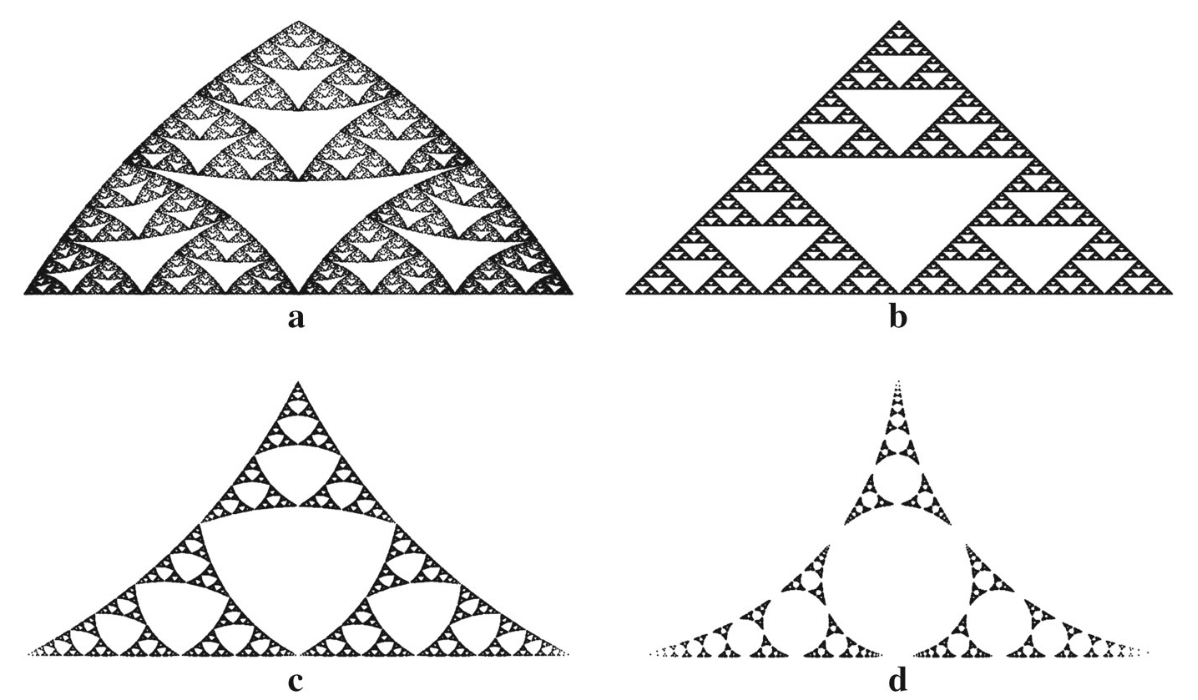
After my re-discovery of the Levitt-Yoccoz gasket in 2006, I got interested in self-projective sets.
These are the natural next step after
self-similar
and self-affine sets but, to my knowledge,
they had never been studied in literature. After a thorough analytical and numerical study of the Levitt-Yoccoz gasket
and its $n$-dimensional
generalization, posted to the arXiv in 2008 but not published elsewhere,
a talk by He Ooh at the University of Maryland in 2011 made me understand the connection
between the Levitt-Yoccoz gasket and the Apollonian gasket and this ultimately led me to prove some of the most important properties
I had seen numerically and failed to prove until then. My results were ultimately submitted in 2013 and published in 2014.
In particular, I proved the results below.
Definition [DL, 2014].
A free semigroup $\cal A=\langle A_1,\dots,A_k\rangle$ of real or complex $n\times n$ matrices is fast
if there is a constant $c>0$ such that
$$
\|M A_i A_j N\|\geq c\|M\|\|A_iA_j N\|
$$
for every $M,N\in\cal A$ and $i\neq j$.
Theorem [DL, 2014].
Let $\cal A$ be a fast free semigroup of $n\times n$ real or complex matrices and let $N(r)$ be the number of elements
of $\cal A$ of norm smaller than $r$. Then
$$
\lim_{r\to\infty}\frac{\log N(r)}{\log r} = s
$$
with $0<s<\infty$.
We call $s$ the exponent of $\cal A$.
Theorem [DL, 2014].
Let ${\cal A}=\langle A_1,\dots,A_k\rangle$ be a fast free semigroup of $2\times 2$ real or complex matrices with exponent $s$ and let
$\Psi=\langle \psi_1,\dots,\psi_k\rangle$ the corresponding semigroup of projective transformations.
Assume that all generators are hyperbolic and that there exist some open set $V$ over which all generators of $\Psi$
are contractions with respect to the Euclidean distance and satisfy
$0<a\leq|\psi'_i|\leq c<1$ for all $i$ and some
constants $a,c$. Finally, assume that the generators satisfy the open set condition $\psi_i(V)\cap\psi_j(V)=\emptyset$.
Let $R\subset V$ be the compact invariant set of $\Psi$. Then $$2\dim_H R=s.$$
An interesting example to which the theorem above applies is given by the 1-paramter family of semigroups of Mobius transformations leaving invariant
the triangle with vertices $-1,i,1$ generated by the three maps $\psi_1,\psi_2,\psi_3$ determined by the following conditions:
$$
\psi_1(1)=1,\;
\psi_1(-1)=0,\;
\psi_1(i)=u+iv
$$
$$
\psi_2(1)=0,\;
\psi_2(-1)=-1,\;
\psi_2(i)=u-iv
$$
$$
\psi_3(1)=u+iv,\;
\psi_3(-1)=u-iv,\;
\psi_3(i)=i,
$$
where $u+iv$ belongs to the circle $x^2+y^2-x=0$.
For each $u\in[1/5,0.651]$, the corresponding invariant compact set $R$ of the semigroup is
a complex self-projective set.

Conjecture [DL, 2014].
Let ${\cal A}\subset SL_n(\mathbb R)$ be a fast semigroup with exponent $s$
and denote by $R$ the invariant compact set of the induced semigroup
of projective transformations. Then
$$
n\dim_H R\geq (n-1)s.
$$
See the articles below for more details, results and examples:
- R. De Leo, Exponential growth of norms in semigroups of linear automorphisms and Hausdorff dimension of self-projective IFS., Journal of Geometrical Analysis, 25:3, 1798-1827, 2015, manuscript
- R. De Leo, A conjecture on the Hausdorff dimension of attractors of real self-projective Iterated Function Systems., Experimental Mathematics, 24:3, 270-288, 2015, manuscript